Solution
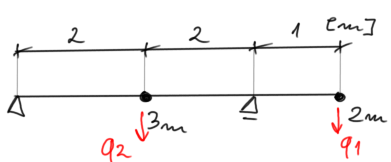
Static indeterminacy degree
SSD=3-3=0Number of dynamic degrees of freedom
LSSD=2
\( m_1=2m, m_2=3m \)
Charts of moments caused by unit forces applied at dynamic degrees of freedom
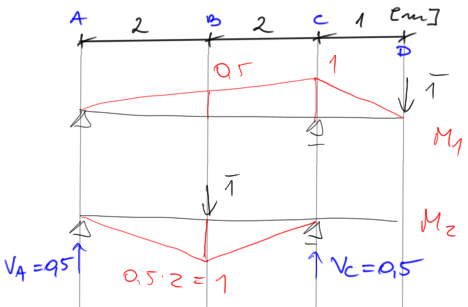
Dynamic deltas
$$ \begin{aligned} &\delta_{11}=\frac{1}{\mathrm{EI}} \cdot\left(\frac{1}{3} \cdot 1 \cdot 1 \cdot 4+\frac{1}{3} \cdot 1 \cdot 1 \cdot 1\right)=\frac{5}{3} \cdot \frac{1}{\mathrm{EI}} \\ &\delta_{22}=\frac{1}{\mathrm{EI}} \cdot\left(\frac{1}{3} \cdot 1 \cdot 1 \cdot 2+\frac{1}{3} \cdot 1 \cdot 1 \cdot 2\right)=\frac{4}{3} \cdot \frac{1}{\mathrm{EI}} \\ &\delta_{12}=\frac{1}{\mathrm{EI}} \cdot\left(\frac{-1}{3} \cdot 0.5 \cdot 1 \cdot 2-\frac{1}{3} \cdot 0.5 \cdot 1 \cdot 2-\frac{1}{6} \cdot 1 \cdot 1 \cdot 2\right)=-1 \cdot \frac{1}{\mathrm{EI}} \end{aligned} $$ Vibration frequencies (note that there are several approaches to calculating vibration frequencies, more in the theoretical introduction - LINK) $$ \begin{aligned} &\mathrm{L}=\mathrm{m}_1 \cdot \delta_{11}+\mathrm{m}_2 \cdot \delta_{22}=\frac{22}{3} \cdot \frac{\mathrm{m}}{\mathrm{EI}} \\ &\mathrm{S}=2 \cdot \mathrm{m}_1 \cdot \mathrm{m}_2 \cdot\left(\delta_{11} \cdot \delta_{22}-\delta_{12}^2\right)=\frac{44}{3} \\ &\omega_1=\sqrt{\frac{\mathrm{L}-\sqrt{\mathrm{L}^2-2 \cdot \mathrm{S}}}{\mathrm{S}}}=0.404 \cdot \sqrt{\frac{\text { EI }}{\mathrm{m}}} \\ &\omega_2=\sqrt{\frac{\mathrm{L}+\sqrt{\mathrm{L}^2-2 \cdot \mathrm{S}}}{\mathrm{S}}}=0.915 \cdot \sqrt{\frac{\text { EI }}{\mathrm{m}}} \end{aligned} $$ After substituting the data \( EI=3.5\cdot 10^5 kNm^2 , m=750kg \) $$ \begin{aligned} &\omega_1=0.404 \sqrt{\frac{3.5 \cdot 10^5 \cdot 10^3}{750}}=275.98 \cdot \frac{\mathrm{rad}}{\mathrm{s}} \\ &\omega_2=0.915 \sqrt{\frac{3.5 \cdot 10^5 \cdot 10^3}{750}}=625.06 \cdot \frac{\mathrm{rad}}{\mathrm{s}} \end{aligned} $$ Vibration amplitudes (more about the approach to calculating vibration amplitudes in the introduction - LINK) $$ A_2=\frac{1-\delta_{11} \cdot m_1 \cdot \omega^2}{\delta_{12} \cdot m_2 \cdot \omega^2} \cdot A_1 $$ assuming \( \mathrm{A}_{11}=1 \quad dla \omega_1=0.404 \sqrt{\frac{\text { EI }}{\mathrm{m}}} \) $$ A_{21}=\frac{1-\frac{5}{3 E I} \cdot 2 \mathrm{~m} \cdot\left(0.404 \sqrt{\frac{E I}{m}}\right)^2}{\frac{-1}{E I} \cdot 3 \mathrm{~m} \cdot\left(0.404 \sqrt{\frac{E I}{m}}\right)^2} \cdot A_{11}=-0.931 $$ assuming \( \mathrm{A}_{12}=1 \quad dla \quad \omega_2=0.915 \sqrt{\frac{\text { EI }}{m}} \) $$ A_{22}=\frac{1-\frac{5}{3 \mathrm{EI}} \cdot 2 \mathrm{~m} \cdot\left(0.915 \sqrt{\frac{\mathrm{EI}}{\mathrm{m}}}\right)^2}{\frac{-1}{\mathrm{EI}} \cdot 3 \mathrm{~m} \cdot\left(0.915 \sqrt{\frac{\mathrm{EI}}{\mathrm{m}}}\right)^2} \cdot \mathrm{A}_{12}=0.713 $$ Orthogonality condition $$ A_{11} \cdot A_{12} \cdot m_1+A_{21} \cdot A_{22} \cdot m_2=0 $$ \( 1 \cdot 1 \cdot 2 m-0.931 \cdot 0.713 \cdot 3 m=0 \)
\( 0.009 m \sim 0 \)
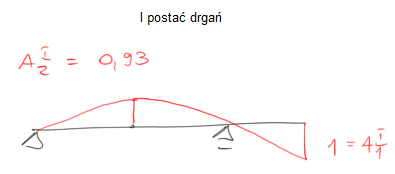
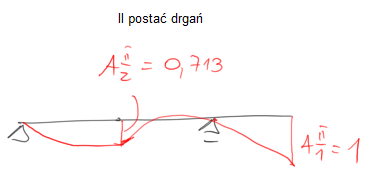
Modes of vibration
If you have any questions, comments, or think you have found a mistake in this solution, please send us a message at kontakt@edupanda.pl.
