Classic Version

Force distribution P into components

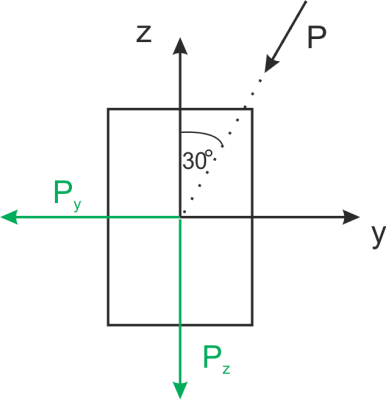\begin{aligned} &P_y=P sinα=5kN\\ &P_y=P cosα=8,66kN\\ \end{aligned}

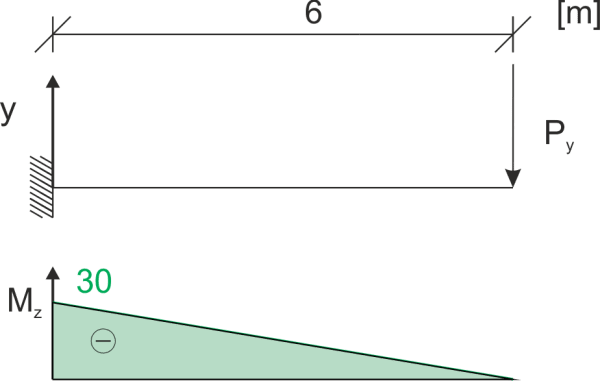
Load in the xz and xy planes, bending moment diagrams

\begin{aligned} &M_y=-P_z⋅6-\frac{1}{2}⋅5⋅6^2=-141,96kNm \end{aligned} \begin{aligned} &M_z=-P_y⋅6=-26\ kNm \end{aligned}

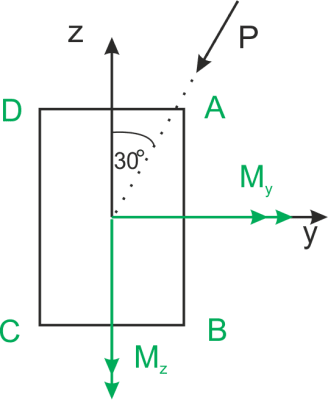
Moment vectors, identification of characteristic section points

Normal stresses

\begin{aligned} &\sigma=\frac{M_y}{I_y}z+\frac{M_z}{I_z}y\\ &I_y=\frac{bh^3}{12}=\frac{2a\cdot\left(3a\right)^3}{12}=\frac{9}{2}a^4\\ &I_z=\frac{hb^3}{12}=\frac{3a\cdot\left(2a\right)^3}{12}=2a^4\\ \end{aligned}

Determining the neutral axis equation

\begin{aligned} &\sigma=0\\ &\sigma_{max}=\frac{141,92\cdot{10}^3}{\frac{9}{2}a^4}\cdot1,5a+\frac{30\cdot{10}^3}{2a^4}\cdot a\\ &z=-0,475y\\ \end{aligned}

Two points are enough to plot a linear function

\begin{aligned} y=0,z=0 y=1,z=-0,475 \end{aligned}

Strength condition σ_max≤k_g

\begin{aligned} &\sigma_{max}=\frac{141,92\cdot{10}^3}{\frac{9}{2}a^4}\cdot1,5a+\frac{30\cdot{10}^3}{2a^4}\cdot a\le k_g\\ &a=0,075m=7,5cm\\ \end{aligned}

Stresses at characteristic points

\begin{aligned} &σ_A=147,72MPa\\ &σ_B=-76,61MPa\\ &σ_C=-147,72MPa\\ &σ_D=76,61MPa\\ \end{aligned

Normal stress diagram