Edupanda » Mechanika budowli » Displacements by Maxwell-Mohr Method » Gerber beams
List of examples
Example 1
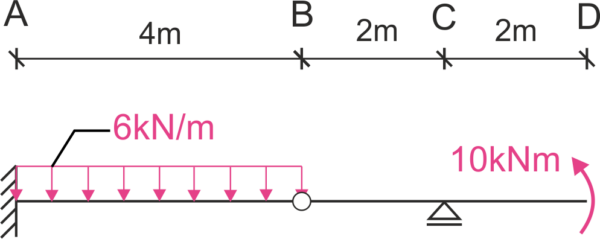
Calculate the vertical displacement of point D, \(\Delta_D.\) Take into account the influence of bending moments and shear forces.
Example 2
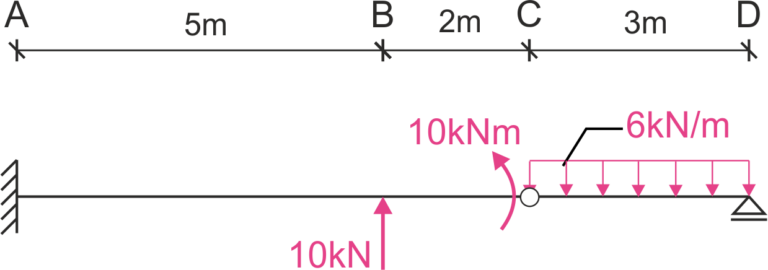
Calculate the rotation angle of point D \(\varphi_D.\) Take into account the influence of bending moments and shear forces.
Example 3

Calculate the vertical displacement of point C \( \Delta_C \), the vertical displacement of point D \( \Delta_D \), the change in angle of rotation of point C \( \Delta\varphi_C \). E=210GPa, I=6500cm^4. Consider only the influence of bending moments.
Example 4
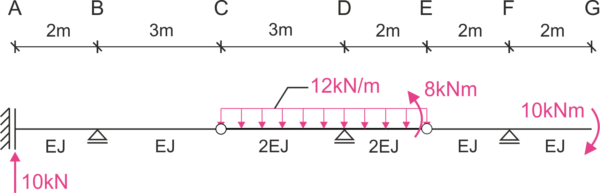
Calculate the vertical displacement of point C \( \Delta_C \), the rotation angle at point G \( \varphi_G \), and the change in rotation angle at point C \( \Delta\varphi_C \). E=210 GPa, I=7500cm^4 Consider only the effects of bending moments.
Example 5
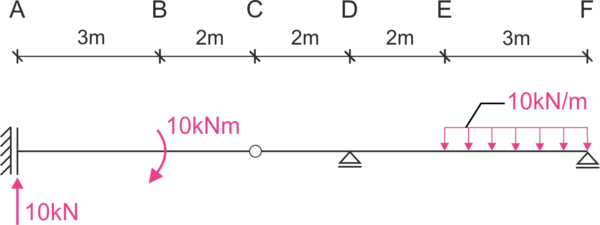
Calculate the vertical displacement of point A \( \Delta_A\) , the vertical displacement of point E \( \Delta_E\) , the rotation angle at point F \( \varphi_F \) and the change in rotation angle at point C \( \Delta\varphi_C.\) Take into account the influence of bending moments. \( E=200 GPa , I=5000cm^4 \)
Example 6
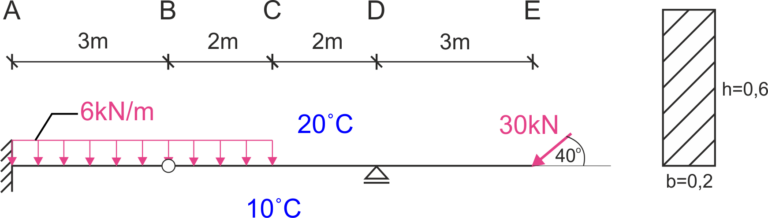
Calculate the vertical displacement of point C (\( \Delta_C\) ), the horizontal displacement of point E (\( \Delta_E\) ), and the change in rotational angle at point B (\( \Delta\varphi_B\)). Take into account the influence of bending moments, shear forces, normal forces, and temperature. Data: \(b=0.2m, h=0.6m, E=205 GPa, \nu=0.3, t_g=20^oC, t_d=10^oC, \alpha=1.2\cdot 10^{-5}, \kappa=1.2\)
Example 7
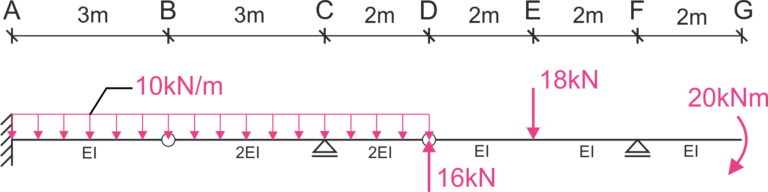
Calculate the rotation angle of node C \(\varphi_C\), vertical displacement of point G \(\Delta_G\), vertical displacement of point D \(\Delta_D\), and change in rotation angle at point D \(\Delta\varphi_D.\) Take into account the influence of bending moments and pay attention to the varied characteristics of cross-sections. \(E=210GPa, I=7650 cm^4\)